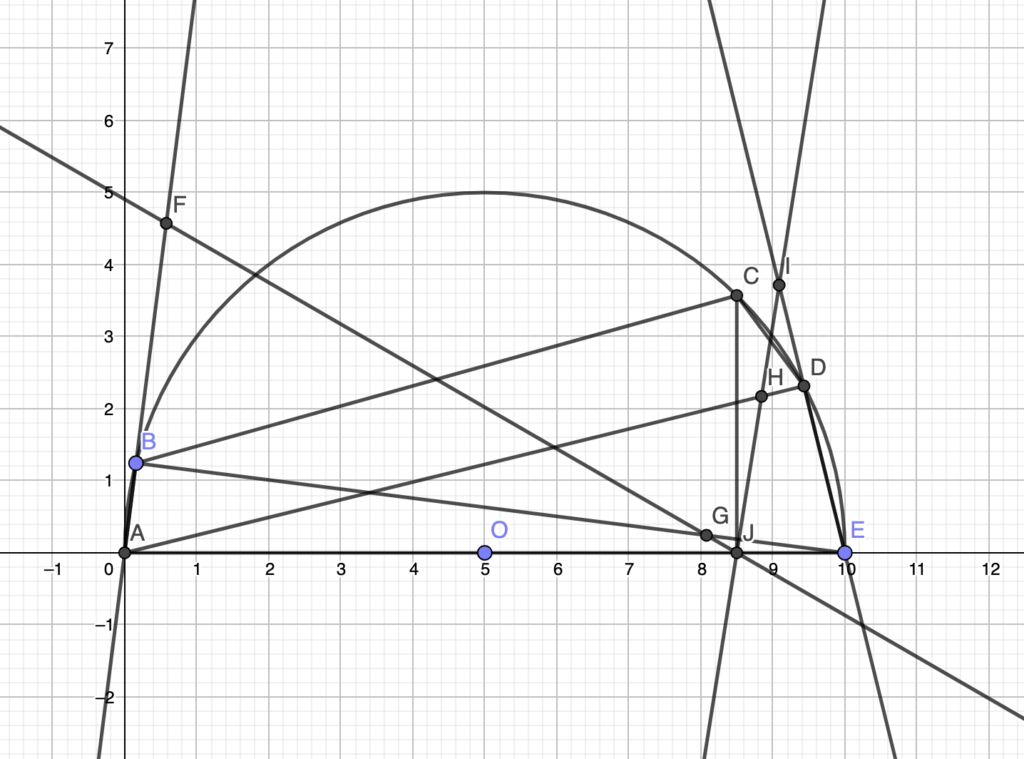
Cyclic Pentagon And Nice Angles – Mattia Giuri's bizarre blog

We have a cyclic pentagon, such that AE is a diameter and equals 10. We know $BC = 5\sqrt{3}$ and $\widehat{COD} = 18$ .

Now F, G, H, I are the feet of the perpendiculars from C to AB, EB, AD, ED. If FG and HI concur at J, find FJI.

Now, let K be the foot of the perpendicular from C to AE. Notice that quadrilaterals CIDH ( $\widehat{CID} = \widehat{CHD} = \frac{\pi}{2}$ ) and CHKA ( $\widehat{CKA} = \widehat{CHA} = \frac{\pi}{2}$ ) are cyclic. Hence, we get:

$\widehat{CHI} = \widehat{CDI} = \pi - \widehat{CDE} = \widehat{CAE} = \pi - \widehat{CHK}$

This means that H, I, K are collinear. By an analogous reasoning, we get that even F, G, K are collinear.

Hence, FG, HI and AE concur at K, which actually is J.

Now, because AFCJ is cyclic ( $\widehat{CFA} = \widehat{CJA} = \frac{\pi}{2}$ ), we get $\widehat{FJC} = \widehat{FAC} = \widehat{BAC}$ .

Similarly, from the ciclicity of CIEJ, we get $\widehat{CJI} = \widehat{CED}$ .

Hence, we deduce $\widehat{FJI} = \frac{\widehat{BOD}}{2}$ .

Because $BD = 5\sqrt{3}$ and the radius of the circumference is 5, we conclude (by the sines theorem) that $\widehat{BOD} = 138$ .

Hence,

$\widehat{FJI} = 69$